Before tuning, it was important that we are able to produce an 'accurate' sound of the required frequency.
This was possible on the Synthesizer (hence, it is called as a 'Synthesizer'). Shadja can be tuned as a starting point to the Internationally specified frequency on the key board. For eg., Key 'A' on a Synthesizer makes a sound of 440 Hz as per the current International specification of keyboard instruments and as per ET tuning.
If we wish to tune 22-Shruti-Harmonium in 'A' or Saphet 6, the procedure to tune B flat or Kali 5 will be as follows.

Figures show the photograph of 22-Shruti-Harmonium with knobs under every key
The mechanical modification of knobs under every key allows any one pair of reeds (with a higher or lower pitch) as required in a Raga to be selected and played.
The player can even do these adjustments while continuing to play the instrument, although this may not be practically necessary.
Selecting a shruti is very simple. When the knobs below the keys are in 'closed' position, all the 'lower' shrutis are 'on'. So the player only has to 'pull' or 'open' the knobs to get 'higher' shrutis as required in a Raga. This is quick and can even be done while playing.
- 1Tuning of S first : Firstly, tune all the 4 reeds under Saphet 6 of 22-Shruti-Harmonium to the sound produced by Key 'A' on the Synthesizer.
- 2Tuning of the lower Shruti on Kali 5 : Next, let us see how to tune the lower Shruti (r1) on the 2 reeds under Kali 5. The 2 reeds (under Kali 5) will have to be tuned to the lower Shruti = 440 x 5.35% = 463.54.
- 3As against this, the key B flat on the synthesizer has a frequency of 440 x 1.0594631 = 466.163764, and firstly we will have to change this sound to 463.54.
- 4The difference in 466.163754 and 440 is = 26.163764. This is further divided into 100 playable parts on the Synthesizer. Thus, each part (called as 'Cent') will be = 26.163764 divided by 100 = 0.26163764. Now, the difference between 463.54 and 440 is 23.54. How many cents of 0.26163764 will be required to fill this difference? 0.26163764 is One cent, so 23.54 is how many cents? 23.54 divided by 0.26173754 = 89.93742357 cents; rounded off to 90 cents.
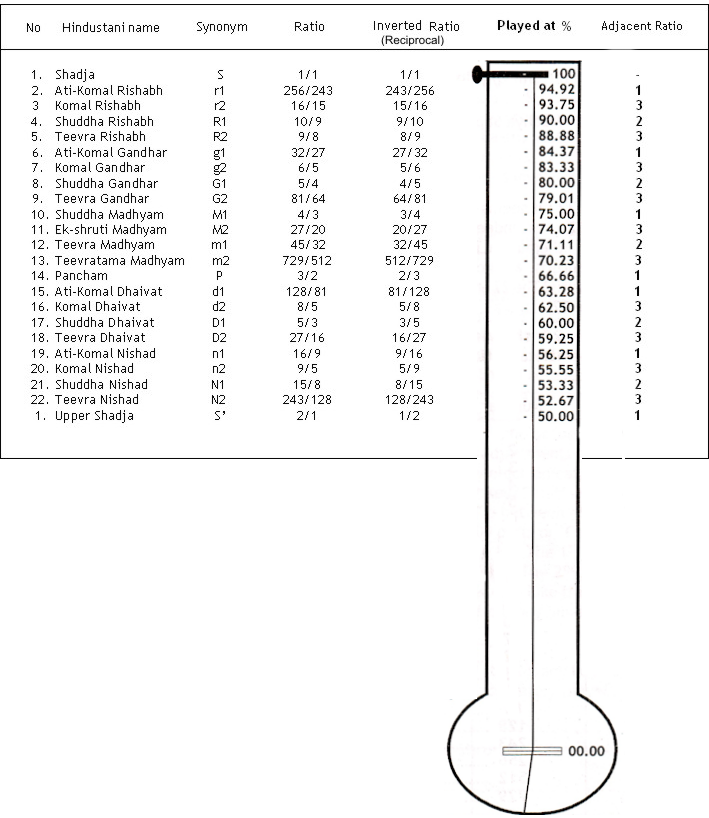
- 5This means that we can produce the sound of (r1) 463.54 Hz by increasing the tone setting on the Synthesizer of Key 'A' by 90 Cents. (Refer to table in Reseach Topic 34)
- 6We do this setting on Key 'A', play the key, and then chisel the 2 reeds under Kali 5 to this sound.
Similarly, the other 2 reeds under Kali 5 will have to be tuned to (r2) the 'higher' Shruti = 440 x 6.66% = 469.304.
This will have to be done by increasing the setting of Key B flat on the Synthesizer by 11, produce the sound and thereafter tuning the remaining 2 reeds under Kali 5 to this sound. Key B flat is on 100 Cents. 111 Cents on the same key will play r2. (Refer to table in Reseach Topic 34.)
Like this, all the 22 Shrutis can be tuned very accurately on 22-Shruti-Harmonium.